Table of Contents
Example: Calculating the velocity of a plane using relative measurements
A Boeing 747 leaves the Detroit airport intending to head due west. The plane is experiencing a strong crosswind that is blowing toward the south, which has a wind speed of 10.0 m/s. Determine the speed of plane relative to the ground and the direction its compass should read if the pilot intends to fly due west at top speed.
Setup
You need to determine the speed and direction of the plane using information given and any information that you can collect or assume.
Facts
- The pilot intends to fly due west.
- The plane experiences a crosswind with a speed of $|v_{a/g}| = 10.0 \dfrac{m}{s}$, which is directed due south.
Lacking
- The top speed of a Boeing 747 is unknown, but can be found online (920 $\dfrac{km}{h}$ or $v_{p/a} = 255 \dfrac{m}{s}$).
Approximations & Assumptions
- The windspeed is measured relative to the ground.
- The plane's airspeed is measured relative to the air.
- At top speed, the plane is flying level with ground.
- The plane has a constant velocity over the interval you care about it.
Representations
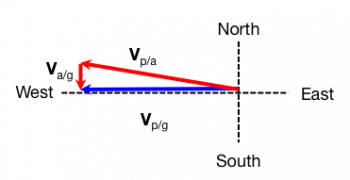
- The velocities of the plane relative to the air, the air relative to the ground, and the plane relative to the ground are represented in the following diagram.
- The relative velocity equation for three objects is: $\vec{v}_{A/C} = \vec{v}_{A/B} + \vec{v}_{B/C}$ where $\vec{v}_{A/C}$ is the velocity of object A with respect to object C, etc.
Solution
The problem can be described vectorially using the relative velocity equation:
$$\vec{v}_{p/g} = \vec{v}_{p/a} + \vec{v}_{a/g}$$
The pilot requires that the velocity of the plane with respect to the ground be directed due west. If you measure positive $\theta$ counterclockwise with respect to the east, the plane's velocity relative to the ground should only have a negative $x$-component. So the equation above becomes (in 2D),
$$\langle -|v_{p/g}|,0 \rangle = |v_{p/a}|\hat{v}_{p/a} + \langle 0, -|v_{a/g}|\rangle$$
We can break this vector equation into two scalar equations:
$$-|v_{p/g}|=|v_{p/a}|{v}_{p/a,x}$$ $$0=|v_{p/a}|{v}_{p/a,y}-|v_{a/g}|$$
where ${v}_{p/a,x}$ and ${v}_{p/a,y}$ are the components of the unit vector in the direction of the velocity of the plane with respect to the air. Thus, they satisfy this equation.
$${v}_{p/a,x}^2 + {v}_{p/a,y}^2 = 1$$
You can rewrite the above equation by using what the unit vector components are equal to (in the previous two equations),
$$\left(-\dfrac{|v_{p/g}|}{|v_{p/a}|}\right)^2+\left(\dfrac{|v_{a/g}|}{|v_{p/a}|}\right)^2= 1$$ $${|v_{p/g}|}^2+{|v_{a/g}|}^2= {|v_{p/a}|}^2$$
So, the speed that the plane has with respect to the ground is slower than its air speed, which agrees with the representation above. $${|v_{p/g}|}= \sqrt({|v_{p/a}|}^2-{|v_{a/g}|}^2) = \sqrt{(255 \dfrac{m}{s})^2 - (10 \dfrac{m}{s})^2} = 225 \dfrac{m}{s}$$
The angle the compass should read can be determined from the above representation. The tangent of the angle (as measured from the negative $x$-axis is given by,
$$\tan \theta = \dfrac{|v_{a/g}|}{|v_{p/g}|}$$
Hence,
$$\theta = \tan^{-1} \left(\dfrac{|v_{a/g}|}{|v_{p/g}|}\right) = \tan^{-1} \left(\dfrac{10 \dfrac{m}{s}}{225 \dfrac{m}{s}}\right) = 2.5^{\circ}$$
which is 2.5$^{\circ}$ north of west or 177.5$^{\circ}$ from east measured counterclockwise.