Suppose you have a two charges, one with value $5 \mu\text{C}$, the other with value $-5 \mu\text{C}$. There are at separate locations, a distance $1 \text{ m}$ apart, and they can be modeled as a dipole. What is the flux through a cylinder with radius $4 \text{ m}$ and length $16 \text{ m}$ that encloses this dipole?

• The dipole charges are $q=5 \mu\text{C}$, $-q=-5 \mu\text{C}$.
• The dipole distance is $1 \text{ m}$.
• The cylinder has radius $4 \text{ m}$ and length $16 \text{ m}$.

• $\Phi_e$ through the cylinder

• The axis of the cylinder is aligned with the dipole.
• The dipole and cylinder are centered with respect to each other.
• The electric flux through the cylinder is due only to the dipole (i.e., no other charges exist).
• The charges are point charges, which indeed means we can model them as a dipole.

• We represent the situation with the following diagram.

First, notice that we probably do not want to do any calculations here, since the it will not be fun to take a dot-product of the dipole's electric field and the area-vector, and it will get very messy very quickly when we start integrating over the surface of the cylinder. Instead, we evaluate the situation more qualitatively. Consider the electric field lines of the dipol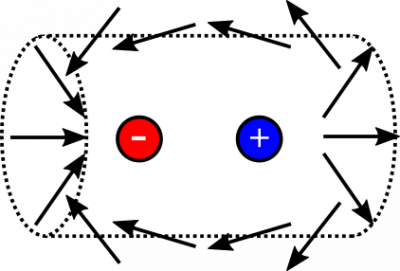e: You can imagine that if we were able to draw this in three dimensions, we would have just as many field lines entering the cylinder as exiting. Since flux is a measure of the “flow” of electric field through a surface, we could say that the flux is zero: the inward flow cancels with the outward flow. For now, we tentatively write: $$\Phi_{\text{cylinder}}=0$$ We gain more confidence when we read the next section of notes, where we define “Gauss' Law”. This law states that the total flux through a close surface is the amount of charge enclosed divided by $\epsilon_0$, the permittivity of free space. $$\Phi_{\text{total}}=\int \vec{E} \cdot \text{d}\vec{A}=\frac{Q_{\text{enclosed}}}{\epsilon_0}$$ Since the total charge of the dipole is $0$, then indeed the charge enclosed is $0$, and we were correct with our reasoning about the electric field and flux above.